Math Intervention

Math intervention provides targeted support to students who are struggling with mathematics concepts and skills. The specific components of a math intervention program can vary depending on the grade level, the needs of the students, and the goals of the program. It’s important to note that the specific design and components of a math intervention program can vary widely based on the educational institution, the grade level of the students, and the resources available. The goal of these programs is to help struggling students fill gaps and reach grade-level proficiency with a solid foundation in mathematics.

Many teachers, parents, and children are unfamiliar with the term dyscalculia, which is often mistakenly called “math dyslexia.” Dyscalculia describes a unique neural condition that affects learning skills related to math or arithmetic. According to research, “The difficulties are not due to a disorder of intellectual development, sensory impairment, mental or neurological disorders, or inadequate instruction (WHO, 2018)” (McCaskey et al., 2020).

Educators commonly call dyscalculia a learning disability, although some researchers believe it is more properly described as a learning difference. The condition was identified in 1919 by Salomon Henschen, a Swedish neurologist, who discovered it during his studies of aphasia and visual pathways of the brain. However, the condition stayed primarily in the province of neurology until the 21st century, when it moved into mathematics education research. It is estimated that dyscalculia affects between 5% and 7% of students and occurs about equally in males and females.

Difficulties common to learners with dyscalculia include:

• Connecting numbers and words that represent numbers
• Recognizing patterns
• Putting things in order
• Learning math facts
• Estimating such things as height, width, or depth
• Adding and subtracting
• Keeping track when counting
• Moving from finger counting to mental counting
• Making sense of charts and graphs

Research shows that the best way to support students with dyscalculia is to present interventions that focus on math learning gaps and that use “multisensory teaching methods, building a child’s confidence, high teacher expectations, and parental support” (PenCRU, 2018).

Although we are still in the early stages of understanding how to support students with dyscalculia, we do know some of the strategies that work: “explicit, repetitive instruction, more time to understand how symbols represent quantity, playful activities to avoid math anxiety, and suggestions for finger counting in more sophisticated ways” (ASCD, 2020). Most of these strategies will benefit all students; they can be used in MTSS and RTI environments. Fortunately for teachers of students who may have dyscalculia, Let’s Go Learn’s system for math education matches the support that ASCD recommends for these students.

Let’s Go Learn’s Dyslexia Screener, DORA DS,  makes it easy for teachers to assess an entire class quickly to identify students who may need reading intervention. Our screener takes most students only 10-15 minutes to complete, and it is automatically scored with reports ready in real time. Students’ dexterity with their devices is measured first to ensure the validity of results.

Click here for a deeper article on Dyscalculia by Margy Hillman

Assessing a student’s math gaps is essential for providing targeted support and intervention. There are several effective ways to assess a student’s math gaps:

• Standardized Tests: Standardized math assessments, such as state-standardized tests or nationally normed tests, can provide a broad overview of a student’s math skills. These tests often cover a range of math topics and can help identify areas where a student is performing below grade level.
• Benchmark Assessments: Benchmark assessments occur at regular intervals to measure mathematics skills during the school year. The first benchmark of the year sets a baseline, and the remaining benchmark assessments measure progress and student achievement.
• Diagnostic Assessments: Diagnostic assessments are designed to pinpoint specific areas of weakness. They are often administered by teachers and can include both written assessments and one-on-one interviews with the student to identify gaps in knowledge and skills.
• Curriculum-based Assessments: Teachers can assess a student’s understanding of specific math concepts by using assessments aligned with the curriculum being taught. These assessments can be administered periodically to track progress.
• Formative Assessment: Formative assessment involves ongoing informal assessments conducted during instruction. Techniques like quizzes, exit tickets, and classroom observations can help identify immediate misunderstandings or gaps as they arise.
• Review of Past Work: Carefully reviewing a student’s previous math assignments, tests, and homework can provide insights into recurring areas of difficulty.
• One-on-One Interviews: Teachers can engage in one-on-one discussions with students to assess their math understanding. This approach allows students to explain their thought processes and reveal areas of confusion.
• Math Portfolios: Collecting a portfolio of a student’s math work over time can provide a holistic view of the student’s math skills, including strengths and weaknesses.
• Peer or Self-Assessment: Sometimes students can assess their own understanding or evaluate their peers’ work. Peer or self-assessment activities can reveal gaps in comprehension when students struggle to explain concepts or provide accurate feedback.
• Math Software and Online Tools: There are various educational software programs and online tools that offer adaptive assessments. These tools can adapt to a student’s level and provide targeted questions to identify gaps.
• Observation and Classroom Performance: Teachers can observe students during math class to assess their participation, engagement, and ability to apply math concepts. Their observations can provide valuable insights into gaps in understanding.
• Parent Input: Parents or guardians can provide valuable information about their child’s math struggles and areas of concern. Collaborating with parents can help in identifying gaps and developing a plan for support.
• Rubrics and Checklists: Teachers can use rubrics or checklists to assess specific math skills or concepts. These tools provide clear criteria for assessment and can help identify gaps in a structured way.

Combining multiple assessment methods can provide a more comprehensive understanding of a student’s math gaps. Once gaps are identified, educators can develop targeted interventions and strategies to help the student catch up and build a solid foundation in mathematics. It’s important to note that ongoing assessment and progress monitoring are key components of effective math instruction and intervention.

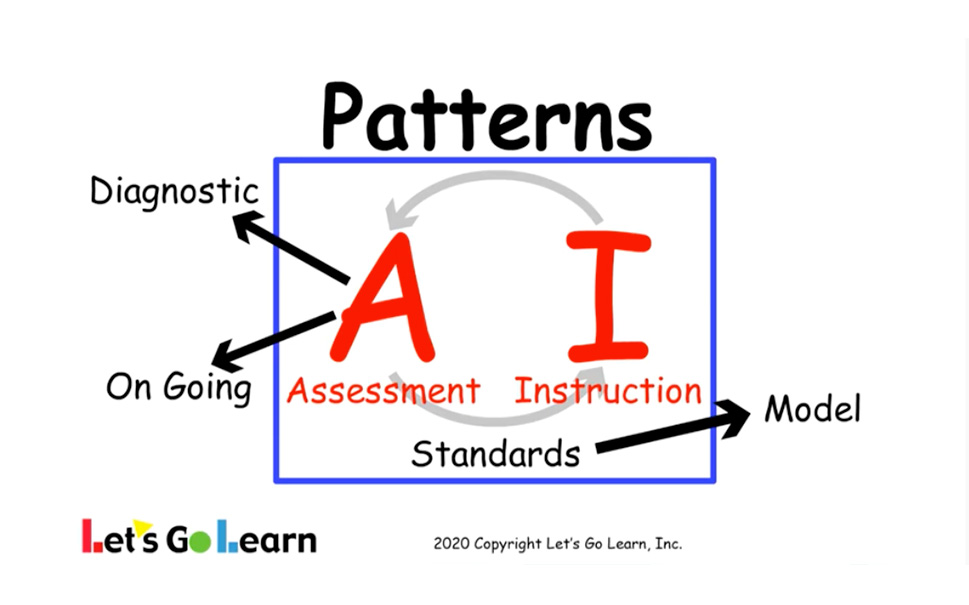
By employing revolutionary adaptive logic, Let’s Go Learn’s math assessments maximize the information obtained about each student while minimizing test-taking time and anxiety. 

The granular data provides educators and teachers with a comprehensive picture of each learner’s strengths and weaknesses at a skill and concept level.  This translates to a prescriptive path for true personalized learning.  For students with IEPs, this means providing automated Specially Designed Instruction (SDI) that both eliminates learning gaps and accelerates learning.

K-12 mathematics education typically covers a wide range of topics and concepts, with the specific content varying somewhat by grade level and educational standards adopted in different regions. However, there are common themes and foundational skills and concepts that are typically taught in K-12 mathematics. Here is a general overview of the content you can expect to see at each grade level:

Kindergarten (K) and Early Elementary (Grades 1-2):

• Counting and cardinality
• Basic addition and subtraction
• Understanding and using numbers up to 100
• Basic shapes and geometry
• Measurement of length, weight, and capacity
• Introduction to data and graphs

Elementary School (Grades 3-5):

• More advanced addition and subtraction, including regrouping
• Multiplication and division concepts and facts
• Fractions and decimals
• Measurement of time, money, and area
• Basic algebraic concepts
• Introduction to probability and statistics

Middle School (Grades 6-8):

• More advanced algebra, including solving equations and inequalities
• Ratios and proportions
• Geometry, including angles, polygons, and basic trigonometry
• Rational and irrational numbers
• Statistics and data analysis
• Probability
• Functions and relations

High School (Grades 9-12):

• Advanced algebra, including quadratic and exponential functions
• Coordinate geometry and advanced trigonometry
• Geometry, including theorems and proofs
• Advanced topics in statistics and probability
• Pre-calculus and calculus
• Advanced topics in number theory
• Linear algebra and matrices (in some curricula)
• Applied mathematics in various fields such as physics, chemistry, and engineering

It’s important to note that the specific curriculum and standards can vary by country and state, so the above list provides a general overview. Additionally, some students may progress through these topics at different rates, and there are often options for advanced or specialized math courses in high school for students with a strong interest in mathematics.

Mathematics pedagogy in the 20th century evolved significantly, reflecting changes in educational philosophies, technological advancements, and research in cognitive psychology and mathematics education. Here are some prominent trends and shifts in math pedagogy during the 20th century.

• Traditional Methods (early 20th century): The early 20th century saw the dominance of traditional teaching methods, often characterized by rote memorization and a focus on procedural skills. Teachers typically used textbooks and lectures as primary instructional tools.
• Progressive Education (1920s-1940s): In response to the traditional methods, progressive educators like John Dewey advocated for more experiential and student-centered approaches. The emphasis shifted toward problem-solving, critical thinking, and active learning.
• New Math Movement (1950s-1960s): The New Math movement aimed to reform math education by emphasizing abstract mathematics, set theory, and formal symbolic logic. While it introduced important mathematical concepts, it was often criticized for being too abstract and disconnected from students’ everyday experiences.
• Back-to-Basics Movement (1960s-1970s): In response to concerns about the perceived shortcomings of New Math, the Back-to-Basics movement emerged, emphasizing a return to traditional methods and a focus on basic arithmetic skills.
• The Calculator Revolution (1970s-1980s): The widespread availability of calculators in the classroom led to a shift in math education. Teachers started incorporating calculators into instruction, allowing students to explore mathematical concepts more deeply.
• Mathematics Education Reform (1980s-present): The 1980s marked the beginning of a significant reform movement in math education, influenced by research on learning and cognitive psychology. Key features include a focus on conceptual understanding, problem-solving, and the use of manipulatives to represent mathematical ideas.
• Standards-based Education (1990s-present): Many countries and states developed mathematics education standards during the 1990s and early 2000s. These standards outline what students should know and be able to do at each grade level. A well known example in the United States is CCSS (the Common Core State Standards).
• Technology Integration (late 20th century-present): The proliferation of computers, software, and the internet has influenced math pedagogy. Technology is now commonly used to enhance math instruction through interactive simulations, online resources, and adaptive learning platforms.

• Inquiry-Based Learning (late 20th century-present): Inquiry-based learning encourages students to explore mathematical concepts through questions, investigations, and discovery. It emphasizes problem-solving, exploration, and mathematical reasoning.
• Cultural and Equity Perspectives (late 20th century-present): Math education has become increasingly sensitive to issues of culture and equity. Efforts have been made to ensure that math instruction is inclusive and culturally relevant to all students.
• Assessment and Data-Driven Instruction (late 20th century-present): The use of assessment data to inform instruction and tailor interventions has become a significant trend. Educators use formative and summative assessments to gauge student progress and adjust teaching accordingly.
• Flipped Classrooms and Blended Learning (21st century): The 21st century has seen the emergence of flipped classrooms, where students learn foundational content at home through videos and engage in more interactive and collaborative activities in class. Blended learning, combining online and in-person instruction, has also gained popularity.

These trends in math pedagogy reflect ongoing efforts to improve math education by incorporating research-based practices, adapting to changes in technology, and addressing the diverse needs of students. Educational approaches continue to evolve as educators seek effective ways to teach mathematics in the 21st century.

Math instruction has seen several trends and shifts in response to advances in technology, changes in educational philosophy, and evolving workforce needs. These trends have aimed to make math education more engaging, accessible, and relevant to students. Here are some key trends in 21st-century math instruction:

• Technology Integration: Technology, including computers, tablets, interactive whiteboards, and educational software, has become integral to math instruction. It allows for interactive simulations, virtual manipulatives, online resources, and personalized learning experiences.
• Flipped Classrooms: The flipped classroom model involves students learning content at home through videos or online resources and then using class time for hands-on activities, collaborative problem-solving, and teacher-guided practice. This approach can promote active learning.
• Project-based Learning (PBL): PBL involves students working on real-world math problems or projects. It encourages critical thinking, collaboration, and application of mathematical concepts to practical situations.
• Problem Solving and Critical Thinking: Math instruction now places greater emphasis on problem-solving skills and critical thinking. Students are encouraged to explore and understand the underlying principles behind math concepts rather than memorizing procedures.
• Data Literacy: In an increasingly data-driven world, math instruction often includes data analysis and interpretation. Students learn to work with data sets, make informed decisions, and communicate their findings effectively.
• Personalized Learning: Adaptive learning platforms and data analytics allow for personalized math instruction tailored to individual students’ needs and learning paces. This approach helps address learning gaps and challenges.
• STEM Integration: Science, Technology, Engineering, and Mathematics (STEM) education often integrates math instruction with other STEM subjects to demonstrate real-world applications and interdisciplinary connections.
• Coding and Computational Thinking: Coding and computational thinking skills are being introduced early in math education. These skills help students understand algorithms, logical reasoning, and problem solving, even in non-programming contexts.
• Math for Equity: Math instruction increasingly focuses on addressing equity gaps by providing opportunities for underrepresented groups and promoting a growth mindset. Culturally responsive teaching practices are emphasized.
• Visual Representations: Visual aids and representations, such as diagrams, graphs, and models, are used to enhance understanding and engage learners. Visual math tools support both concrete and abstract mathematical concepts.
• Global Competence: Math education aims to prepare students for a globalized world by exposing them to international math standards and perspectives, and cross-cultural problem solving.
• Online and Blended Learning: The growth of online and blended learning environments has allowed students to access math instruction beyond the traditional classroom. Virtual classrooms and remote learning platforms have become more common.
• Assessment and Data-driven Instruction: Continuous assessment and data analysis help teachers monitor student progress and adjust instruction accordingly. Formative assessment strategies inform instructional decisions in real-time.
• Financial Literacy: Math instruction increasingly includes financial literacy components, helping students understand personal finance, budgeting, investing, and economic concepts.
• Accessible Education: Efforts are made to ensure that math instruction is accessible to all students, including those with disabilities. This includes providing accommodations and accessible materials.
• Science of Math: Similar to the Science of Reading, this movement seeks to establish a research base composed of concepts, procedures, strategies, reasoning, and dispositions. The basic premise is that math proficiency is a basic educational right.

These trends reflect a broader shift in math education toward student-centered, inquiry-based, and technology-enhanced learning experiences that prepare students for the demands of the 21st century. They aim to foster mathematical understanding, critical thinking, problem-solving skills, and the ability to apply math in diverse contexts.

The Common Core State Standards (CCSS) for Mathematics, often referred to as the Common Core Math Standards, are a set of K-12 educational standards for mathematics in the United States. Developed by a consortium of states, these standards were designed to provide a clear and consistent framework for what students should learn in mathematics at each grade level, ensuring that they are well prepared for college and career. Here is a brief description of the key features and components of the Common Core Math Standards:

• Grade-level Expectations: The Common Core Math Standards specify what students are expected to learn in mathematics at each grade level, from Kindergarten through grade 12. The standards are organized into grade clusters: K-2, 3-5, 6-8, and high school.
• Focus on Fewer Topics: One of the distinctive features of the Common Core Math Standards is the focus on a smaller number of key topics or concepts at each grade level. The intention is to promote a deeper understanding and mastery of fundamental mathematical principles.
• Coherence and Progression: The standards are designed to be coherent and build upon each other as students progress through the grades. They aim to ensure that students develop a strong foundation in mathematics and are prepared for more advanced concepts.
• Mathematical Practices: In addition to content standards, the Common Core Math Standards include a set of eight mathematical practices that describe the behaviors and habits of mind that proficient math students exhibit. These practices emphasize problem solving, reasoning, and mathematical communication.
• Real-world Application: The standards emphasize the importance of connecting mathematical concepts to real-world situations, modeling, and problem solving. This approach helps students see the practical applications of mathematics in various fields and careers.
• Rigorous and Conceptual Understanding: The Common Core Math Standards stress the importance of students not only knowing how to perform mathematical procedures but also understanding why these procedures work. This promotes a deeper conceptual understanding of mathematics.
• Assessment Alignment: The standards are often accompanied by aligned assessments, including both formative assessments (to gauge student understanding during instruction) and summative assessments (to measure overall proficiency). These assessments are designed to reflect the standards’ focus on conceptual understanding and problem solving.
• Flexibility for Educators: While the standards provide a clear outline of what should be taught at each grade level, they also allow flexibility for educators to design instructional approaches and materials that best meet the needs of their students.

It’s important to note that over time, each state has customized the original standards, although the key elements have changed very little.

State-customized versions of CCSS are often referred to as “state-specific adaptations” or “state-added standards.” Here’s how states have typically customized the CCSS:

• Additional Standards: Some states added standards that were not originally included in the CCSS. These additional standards might cover specific content areas, skills, or topics that the state deemed important. For example, a state might add standards related to state history or local geography.
• Modifications to Language: States have sometimes modified the language of the CCSS to make them more accessible or to clarify certain concepts. These modifications could include rewording or simplifying standards for different grade levels.
• Reordering or Combining Standards: States might rearrange the order of standards or combine multiple standards into a single standard. This can be done to better align with the state’s curriculum or instructional approach.
• Grade-level Adjustments: In some cases, states have adjusted the grade level at which certain standards are introduced. For example, a state might decide to introduce a specific mathematical concept in a different grade than what is outlined in the original CCSS.
• Deletions: States may choose to remove specific standards or topics from the CCSS that they do not consider essential or relevant to their educational goals, which allows for a more streamlined curriculum.
• Additional Resources: States often provide additional guidance, resources, or sample materials to help educators implement the standards effectively. These resources may include curriculum frameworks, model lessons, and professional development materials.
• Assessment Alignment: States that have their own standardized assessments may align these assessments with their customized versions of the CCSS. This ensures that the tests reflect the specific content and skills emphasized in the state’s standards.

It is important to note that not all states made significant modifications to the CCSS. Some states adopted the standards with minimal changes, while others made more extensive alterations to better suit their educational goals and local contexts. The degree of customization varied from state to state.

A number of states now require math support for struggling students. These include the following states:

• Alabama. 2022. SB 171
• Arkansas. 2023. SB 294
• Colorado. 2023. HB23-1231
• Florida. 2023. HB 7039
• Louisiana. 2023. SB 163
• Virginia. 2022. HB 938
• West Virginia. 2023. HB 3035

There are some states that never approved the original CCSS. These states either chose not to adopt the CCSS at all or had previously adopted them but later repealed or replaced them with state-specific standards. Some of the states with non-Common Core standards include:

• Texas: Texas was one of the earliest states to reject the Common Core standards. Instead, they have their own set of standards called the Texas Essential Knowledge and Skills (TEKS) for various subjects, including mathematics and English language arts.
• Alaska: Alaska chose not to adopt the Common Core standards and developed its own Alaska Content and Performance Standards for English Language Arts and Mathematics.
• Oklahoma: Oklahoma initially adopted the CCSS but later repealed them and replaced them with the Oklahoma Academic Standards for English Language Arts and Mathematics.
• Nebraska: Nebraska never formally adopted the Common Core standards. Instead, they have their own state standards for education.
• Virginia: Virginia originally adopted the CCSS but later made modifications to create the Virginia Standards of Learning (SOL) in English, mathematics, science, and history.
• Indiana: Indiana initially adopted the Common Core standards but later withdrew from the initiative and replaced the standards with their own Indiana Academic Standards.
• South Carolina: South Carolina initially adopted the CCSS but later withdrew from the standards and replaced them with the South Carolina College and Career-Ready Standards.
• Missouri: Missouri initially adopted the Common Core Standards but later reviewed and revised them to create the Missouri Learning Standards for English Language Arts and Mathematics.

It’s important to note that education policies can change, and states may revisit their standards over time. Therefore, the status of standards in each state will continue to evolve.

Math intervention school programs can play a crucial role in promoting equity and inclusion in education by addressing the diverse needs of all students and ensuring that every student has an opportunity to succeed in mathematics. Here’s how math intervention programs can provide equity and inclusion:

• Identifying and Addressing Individual Needs: Math intervention programs typically begin with assessments to identify students who are struggling with specific math concepts or skills. This personalized approach allows educators to understand each student’s unique needs and tailor instruction accordingly. By recognizing and addressing individual learning gaps, intervention programs can help all students, regardless of their starting point.
• Differentiated Instruction: Math intervention programs often involve small-group instruction or one-on-one support, which allows teachers to differentiate their instruction to meet the diverse needs of students. This approach ensures that struggling learners receive targeted support while advanced learners can be challenged appropriately.
• Culturally Responsive Teaching: Equity in education includes acknowledging and respecting students’ cultural backgrounds and experiences. Math intervention programs can incorporate culturally relevant materials and teaching strategies to make math instruction more inclusive and relatable to all students.
• Language Support: For English language learners (ELLs) and students with language-related challenges, math intervention programs can provide language support to help students better understand math concepts. This may involve using visuals, manipulatives, or bilingual resources.
• Access to Resources and Technology: Equity in education also involves ensuring that all students have access to necessary resources. Math intervention programs can provide access to technology, educational software, and materials that may not be readily available to students at home.
• Special Education Services: Math intervention programs can be designed to accommodate students with disabilities by providing specialized instruction and accommodations, such as extra time, assistive technology, or alternative assessments.
• Family and Community Engagement: Math intervention programs can involve parents and caregivers in the learning process, helping them understand their child’s progress and providing strategies for support at home. Engaging families and the community fosters a sense of inclusion and shared responsibility for student success.
• Inclusive Curriculum and Materials: Math intervention programs can select or develop curriculum materials that reflect diverse perspectives and experiences. This helps students see themselves represented in the learning materials and promotes a more inclusive learning environment.
• Teacher Professional Development: Equipping teachers with the skills and knowledge to effectively teach a diverse student population is essential. Professional development opportunities that focus on culturally responsive teaching, inclusive practices, and differentiated instruction can enhance equity in math education.
• Monitoring and Accountability: It is essential to regularly assess and monitor the effectiveness of math intervention programs in order to ensure that they are indeed promoting equity and inclusion. Data analysis can help identify disparities and guide program improvements.
• Positive Learning Environment: Creating a positive and supportive learning environment is crucial for equity and inclusion. Math intervention programs can emphasize the importance of a growth mindset, celebrate diverse perspectives, and encourage collaboration among students.

By addressing the individual needs of students, providing targeted support, and creating an inclusive and equitable learning environment, math intervention programs can help close achievement gaps and ensure that all students have the opportunity to excel in mathematics.

Math intervention programs focus on addressing gaps in students’ mathematical understanding and helping them build a strong foundation in key math topics. The specific topics covered in math intervention programs may vary based on the grade level of the students and their individual needs. However, here are some key math topics that are often addressed in math intervention programs:

Foundational Arithmetic Skills (K-5):

• Number recognition and counting
• Place value and understanding the base-ten system
• Addition and subtraction facts and strategies
• Multiplication and division facts and strategies
• Understanding fractions, including equivalence and basic operations
• Measurement concepts, including length, weight, volume, and time
• Basic geometry, including shapes, angles, and symmetry
• Data and graph interpretation
• Problem-solving skills with a focus on real-life contexts

Operations and Algebraic Thinking (3-8): 

• Solving one-step and multi-step word problems
• Applying addition, subtraction, multiplication, and division to solve problems
• Understanding properties of operations
• Identifying and generating patterns and rules
• Solving equations and inequalities

Number and Operations (3-8):  

• Fractions, decimals, and percents, including operations with fractions.
• Place-value understanding in decimal numbers
• Rational and irrational numbers
• Understanding and working with positive and negative integers
• Estimation and rounding
• Prime and composite numbers, factors, and multiples

Measurement and Data (K-8): 

• Measurement conversions and units.
• Data representation, including tables, graphs, and charts
• Probability and statistics concepts
• Measurement of area and volume
• Time, money, and measurement of angles

Geometry (K-8): 

• Geometric shapes and properties.
• Measurement of geometric figures
• Spatial reasoning and transformations

Ratios and Proportional Relationships (6-8):

•  Ratios and rates.
• Proportional relationships and understanding proportions
• Scale drawings and models

Statistics and Probability (6-8): 

• Data analysis and interpretation.
• Probability concepts, including probability models

Algebra (6-8 and high school):  

• Linear equations and inequalities.
• Graphing linear equations
• Systems of equations
• Exponents and exponential functions
• Introduction to quadratic functions (in higher-level intervention programs)

It’s important to note that math intervention programs are designed to address the specific needs of students, so the selection of topics and the depth of coverage can vary. These programs typically begin by assessing students’ math skills to identify gaps and then provide targeted instruction and practice in areas where students are struggling the most. As students progress, the intervention may extend to more advanced topics based on students’ readiness and needs.

Effective math intervention strategies have been the subject of extensive research in the field of education. Several research studies have investigated approaches and methods to support students who are struggling with mathematics. Some influential studies and research-based strategies that have garnered attention for their effectiveness in math intervention are described below.

RTI (Response to Intervention) and MTSS (Multi-Tiered System of Supports) are both educational frameworks that provide structured approaches to supporting students who may be struggling with academic skills, including math. While there is overlap between the two, there are also key differences in their focus and scope. 

Here’s a breakdown of the main differences between RTI and MTSS math intervention:

Focus

• RTI (Response to Intervention): RTI primarily focuses on identifying and providing interventions for students who are struggling academically. It is often associated with academic interventions to address specific learning difficulties, including those related to math. RTI is more narrowly focused on academic response and progress.
• MTSS (Multi-Tiered System of Supports): MTSS is a broader framework that encompasses academic, behavioral, and social-emotional supports for all students. While it includes academic interventions like RTI, it also addresses a wide range of student needs, including behavior, attendance, and social-emotional well-being.

Tiers

• RTI: RTI typically consists of three tiers. Tier 1 is universal instruction (core curriculum) for all students, Tier 2 is targeted intervention for students at risk, and Tier 3 is intensive intervention for students with significant learning difficulties.
• MTSS: MTSS incorporates multiple tiers, but it can include more than three. While academic interventions are an essential component, additional tiers are included for behavior, social-emotional learning, and other areas of support.

Comprehensive Approach

• RTI: RTI is primarily focused on academic interventions, and its primary goal is to improve academic outcomes for students. It may not address other areas of student well-being or support services.
• MTSS: MTSS takes a more holistic approach, recognizing that students’ needs go beyond academics. It addresses the whole child and provides a framework for schools to coordinate and integrate various support services.

Data Utilization

• RTI: RTI relies heavily on academic data, such as assessments and progress monitoring, to identify struggling students and guide intervention decisions. It is primarily data driven and focuses on academic response to interventions.
• MTSS: While MTSS uses academic data, it also incorporates non-academic data, such as behavioral and social-emotional data, to provide a more comprehensive understanding of a student’s needs. This broader range of data informs decisions about the appropriate tier of support.

Collaborative Team Approach

• RTI: RTI often involves educators and specialists working together to design and implement academic interventions. It may not always include a full team approach that addresses all aspects of a student’s well-being.
• MTSS: MTSS emphasizes a collaborative team approach that may include educators, counselors, behavioral specialists, and other professionals to address different student needs.

In summary, while both RTI and MTSS involve tiered interventions and data-driven decision making, RTI is more focused on academic responses to intervention, while MTSS is a more comprehensive framework that addresses a broader range of student needs, including academic, behavioral, social-emotional, and wellness supports. MTSS is designed to promote a holistic approach to supporting the well-being and success of all students.

For students with disabilities or students at any MTSS/RTI Tier, LGL’s progress-monitoring process consists of five steps: 1) LGL diagnostics analyze and identify each student’s learning gaps and present levels (PLAAFP); 2) IEP teams develop individualized education programs (IEPs) using LGL narratives, diagnostic data, and alignments to standards; 3) The LGL system assigns specially designed instruction (SDI) for students with disabilities and places them in lessons at their zone of proximal development. Teachers can always self-select lesson assignments; 4) LGL provides progress monitoring with pre-built skills and concepts quizzes and tests. Teachers queue assessments, and data automatically overlay onto the original dataset; 5) LGL math curriculum also provides ongoing progress monitoring, tracking student academic performance from the initial evaluation. Teachers are not required to collect data, so they can concentrate on interpreting and implementing mathematics instruction.

Mathematics Recovery is an evidence-based intervention program designed to provide targeted support to students who struggle with mathematics. This program is primarily aimed at early elementary school students but can be adapted for older students as well. Math Recovery was developed by Dr. Robert J. Wright and his colleagues and is based on research in mathematics education and child development. Here are some key features and components of the Math Recovery Program:

• Individualized Assessment: The program begins with a thorough, individualized assessment of each student’s mathematical understanding. Specially trained Math Recovery specialists conduct these assessments to identify specific gaps in a student’s mathematical knowledge and skills.
• One-on-one Instruction: Math Recovery sessions typically involve one-on-one instruction or instruction in very small groups. This personalized approach allows the Math Recovery specialist to tailor the intervention to the student’s unique needs.
• Concrete-Pictorial-Abstract Approach: Math Recovery emphasizes the use of concrete materials, pictorial representations, and abstract symbols to help students understand mathematical concepts. This approach aligns with research on how young children develop mathematical understanding.
• Focus on Number Sense: Number sense, which includes an intuitive understanding of numbers, quantities, and relationships, is a central focus of Math Recovery. Students work on building a strong foundation in number sense to support their overall mathematical development.
• Developmental Progression: Math Recovery is designed to follow a developmental progression, gradually building upon students’ prior knowledge and skills. It starts with foundational concepts and then moves to more advanced topics.
• Problem Solving: The program emphasizes problem solving and critical thinking skills, encouraging students to think deeply about mathematical concepts and apply their understanding to math problems.
• Regular Progress Monitoring: Math Recovery specialists regularly monitor and assess students’ progress to determine whether the intervention is effective. Adjustments are made based on ongoing assessment data.
• Professional Development: Math Recovery specialists undergo specialized training to implement the program effectively. They learn how to conduct assessments, design interventions, and work with students to build mathematical understanding.
• Parent and Teacher Involvement: Math Recovery often involves collaboration with classroom teachers and parents or caregivers. Teachers receive guidance on how to support students in the classroom, and parents may be provided with resources to reinforce learning at home.
• Research-based: The Math Recovery Program is grounded in mathematics education and child development research. It draws on best practices in math instruction and intervention.

Math Recovery is known for its success in helping struggling students build a strong mathematical foundation and catch up with their peers. It is often used in early elementary grades to address gaps in number sense and basic math skills. However, the principles of Math Recovery can also be applied to supporting older students who are struggling with math.

The National Center on Intensive Intervention (NCII) provides resources and guidance on evidence-based practices and intervention strategies in education, including mathematics. NCII focuses on helping educators implement interventions effectively to support students with diverse learning needs, including those who are struggling in mathematics. While NCII does not endorse specific intervention programs, it does promote the use of evidence-based practices. Here are some key principles and strategies often associated with NCII’s approach to math intervention:

• Data-driven Decision Making: NCII emphasizes the importance of collecting and analyzing data to inform intervention decisions. This process includes using data to identify students needing support, monitor their progress, and adjust instruction as necessary.
• Screening and Assessment: NCII recommends using screening tools and assessments to identify students who may be at risk of experiencing math difficulties. These assessments help educators pinpoint specific areas of need and determine appropriate interventions.
• Tiered Intervention Model: NCII promotes the use of a multi-tiered system of supports (MTSS) or response-to-intervention (RTI) framework, which includes three tiers of support. Tier 1 provides high-quality, universal instruction for all students, while Tiers 2 and 3 offer increasingly intensive interventions for students who require additional support.
• Evidence-based Instructional Practices: NCII encourages the use of evidence-based instructional practices in math interventions. These practices are grounded in research and have been shown to be effective in improving student outcomes. Examples include explicit instruction, visual representations, and systematic instruction.
• Progress Monitoring: Educators are encouraged to use ongoing progress monitoring to assess whether interventions are working and to make data-driven decisions about whether to adjust or intensify instruction. Progress-monitoring tools and assessments are typically used.
• Individualized Instruction: NCII recognizes that students have unique needs, and interventions should be tailored to each student’s specific difficulties and strengths. Individualized instruction ensures that interventions are matched to the student’s level of readiness and need.
• Fidelity of Implementation: To ensure that interventions are delivered as intended, NCII emphasizes the importance of fidelity of implementation. This means that educators should follow the intervention program with fidelity, adhering to the recommended procedures and strategies.
• Collaboration: Collaborative teamwork among educators, specialists, and support staff is essential in implementing effective math interventions. Collaboration facilitates data sharing, decision making, and coordination of support services.
• Professional Development: NCII supports ongoing professional development for educators to ensure that they have the knowledge and skills necessary to implement evidence-based math interventions effectively.
• Family Engagement: Involving parents and caregivers in the intervention process is considered crucial. Educators should communicate with families, share progress data, and provide resources for supporting the child’s math development at home.
• Cultural Responsiveness: NCII acknowledges the importance of culturally responsive practices in intervention. Educators should consider students’ cultural backgrounds and experiences when designing and implementing interventions.

It’s important to note that while NCII provides resources and guidance on math interventions, the specific intervention program or strategy may vary by school, district, or individual student needs. Educators often select or design interventions based on the best available evidence and the unique needs of their students. The overarching goal is to ensure that all students receive the support they need to succeed in mathematics.

LGL Math Edge provides a research-based, data-driven, systematic math curriculum that increases student math abilities across any mathematics initiative. Lessons are highly effective and diagnostically driven. They close gaps, boost students’ math skills and concepts, and can be used as supplemental SDI for students with IEPs. Teachers can use the lessons at math centers, for pull-out interventions, or for general classroom mathematics instruction. For schools using MTSS or RTI, scaffolded lessons are automatically generated for every tier.

Math anxiety refers to a feeling of apprehension or fear when it comes to mathematics. This anxiety can have significant consequences for students, both in terms of their academic performance and overall well-being. Here are some of the potential consequences of math anxiety:

• Poor Academic Performance: Math anxiety can lead to a decrease in math grades and overall academic performance. Students who are anxious about math often struggle to concentrate and perform poorly on tests and assignments.
• Avoidance of Math: Individuals with math anxiety may actively avoid math-related tasks and courses, which can limit their educational and career opportunities. This avoidant behavior can perpetuate the problem, as they miss out on opportunities to improve their math skills.
• Negative Self-Perception: Math anxiety can erode an individual’s self-confidence and self-esteem. When students consistently struggle with math, they may start to doubt their abilities in other areas as well, leading to a negative self-image.
• Reduced Career Options: Math skills are essential in a wide range of professions, including STEM fields, finance, and many others. Math anxiety can limit career choices and opportunities for individuals who are otherwise capable but are held back by their fear of math.
• Emotional Distress: Math anxiety can lead to emotional distress, including feelings of frustration, helplessness, and even depression. The constant worry about math-related tasks can take a toll on a person’s mental and emotional health.
• Physical Symptoms: Some individuals may experience physical symptoms of anxiety, such as increased heart rate, sweating, and nausea when faced with math-related tasks or tests.
• Decreased Problem-solving Skills: Math anxiety can interfere with a person’s ability to think logically and solve problems, not just in math but also in everyday situations that require critical thinking and analytical skills.
• Limited Financial Literacy: Math anxiety can hinder a person’s ability to manage finances effectively, as many financial tasks involve basic mathematical concepts. This can lead to financial difficulties in the long run.
• Impact on Parenting: Parents with math anxiety may struggle to help their children with their math homework or provide them with the necessary support and encouragement to excel in math-related subjects.
• Long-term Effects: Math anxiety can persist into adulthood, affecting one’s personal and professional life. It may limit career advancement and reduce opportunities for lifelong learning.

It’s important to note that math anxiety is a real and common issue, and individuals experiencing it should seek help and support. There are strategies and techniques, such as cognitive-behavioral therapy, tutoring, and relaxation exercises, that can help individuals manage and overcome math anxiety. Early intervention and support can prevent the long-term consequences associated with math anxiety and allow individuals to develop a more positive relationship with mathematics.

To ensure that all students reach optimal levels of achievement in mathematics learning, districts and schools must create an inclusive learning environment that accommodates learning differences. Here are some key aspects of inclusivity in math intervention:

• Identifying Diverse Needs: Inclusivity begins with recognizing the diverse needs of students. Some students may require additional support in math due to learning disabilities, language barriers, or other factors. Others may need advanced enrichment to stay engaged and challenged.
• Differentiated Instruction: Inclusive math intervention programs should employ differentiated instructional techniques. This means tailoring instruction to meet the individual needs of each student. For example, teachers can provide alternative ways to explain math concepts, offer extra practice for struggling students, or develop enrichment activities for advanced learners.
• Cultural Sensitivity: Math intervention programs should be culturally sensitive and take into account the diverse cultural backgrounds of students. This includes recognizing that different cultures may have their own approaches to math and respecting those differences.
• Accessibility: Ensure that math materials, resources, and technology used in interventions are accessible to all students. This includes making accommodations for students with disabilities and ensuring that materials are available in multiple formats, such as Braille, large print, or audio.
• Language Support: For students who are English language learners (ELLs), math interventions should provide language support. This might include bilingual instruction or additional language assistance to help ELLs understand math concepts.
• Positive Learning Environment: Create a positive and inclusive classroom or learning environment where all students feel valued, respected, and safe to ask questions and make mistakes. This can help reduce anxiety and foster a love for math.
• Teacher Training: Provide professional development and training for teachers and intervention specialists so they are equipped to implement inclusive math interventions effectively. This training should include strategies for working with diverse student populations.
• Regular Assessment and Monitoring: Continuously assess and monitor the progress of students in math interventions in case it becomes necessary to make adjustments to the support being provided. This ensures that interventions are effective and tailored to individual needs.
• Parent and Community Involvement: Involve parents and the community in the math intervention process. Collaboration among teachers, parents, and the community can help provide additional support and resources for students.
• Flexible Timelines: Recognize that students may progress at different rates in math intervention programs. Allow for flexibility in timelines and avoid rigid expectations for when students should achieve specific milestones.

Inclusive math intervention not only helps students with diverse needs succeed in mathematics but also promotes a more equitable and accessible education system. Schools and educators must be proactive in identifying and addressing the unique needs of all students to ensure that they have equal opportunities to excel in math.

Council for Exceptional Children (CEC). (2019). CEC position on specialized mathematics instruction for students with disabilities.

Gersten, R., & Chard, D. J. (2001). Number sense: Rethinking arithmetic instruction for students with mathematical disabilities. The Journal of Special Education, 35(1), 40-48.

Ginsburg, H. P. (2015). Math anxiety: What is known and what is still to be understood. In S. A. Dowker (Ed.), Individual differences in arithmetic: Implications for psychology, neuroscience, and education (pp. 315-327). Psychology Press.

Hattie, J. (2017). Visible learning: A synthesis of over 800 meta-analyses relating to achievement. Routledge.

Jitendra, A. K., & Star, J. R. (2012). Mathematical word problem solving: A review of cognitive and metacognitive processes. Remedial and Special Education, 33(5), 299-309.

Klein, D. (2003). A brief history of American K-12 mathematics education. Ed. James Royer. Information Age Publishing. http://www.csun.edu/~vcmth00m/AHistory.html

National Center on Intensive Intervention. Intensive mathematics intervention: Instructional strategies. https://intensiveintervention.org/instructional-strategies-math-course 

National Council of Teachers of Mathematics (NCTM). (2014). Principles to actions: Ensuring mathematical success for all. Reston, VA: NCTM.

National Council of Teachers of Mathematics (NCTM). (2016). Catalyzing change in high school mathematics: Initiating critical conversations. Reston, VA: NCTM.

Rivera, D., & Rice, M. (2018). Mathematics intervention: Strategies to support struggling students. National Council of Teachers of Mathematics.

Szűcs, D. (2019). The origins of math anxiety and interventions. IBE – UNESCO. https://solportal.ibe-unesco.org/articles/the-origins-of-math-anxiety-and-interventions.

Tomlinson, C. A., & Imbeau, M. B. (2010). Leading and managing a differentiated classroom. ASCD.

Tobias, S. (1993). Overcoming math anxiety. W. W. Norton & Company.

Witzel, B. S., Mercer, C. D., & Miller, S. P. (2003). Teaching mathematics meaningfully: Solutions for reaching struggling learners. Routledge.

Margy Hillman is an experienced educator and writer who develops learning experiences and products that engage the brain and trigger creative and critical thinking. As part of the Let’s Go Learn team, she studies the education environment and learning research, trends, and strategies, documenting the role of Let’s Go Learn products in transforming learning loss into learning gain. She has a BA in English and an MA in American Studies and K-12 and adult teaching credentials. In addition to her work with K-12 teachers and learners, she is an adjunct professor at National University in Strategic Communications.